Discovering Group Theory, Day 6

On Day 6, we dove into dihedral group D₃, which students have been exploring independently as part of their homework and in the context of the symmetries of an equilateral triangle.

• We tackled the group using three methods:
  • physically manipulating a cutout of an equilateral triangle,
  • drawing diagrams of various operations, simple (single rotation or reflection) and combined (rotation followed by a reflection, etc.), and
  • working out the results of combined operations algebraically.This was an attempt to unify the physical experience of manipulating an object, visually representing the effects of those manipulations on the object, and expressing such operations symbolically.

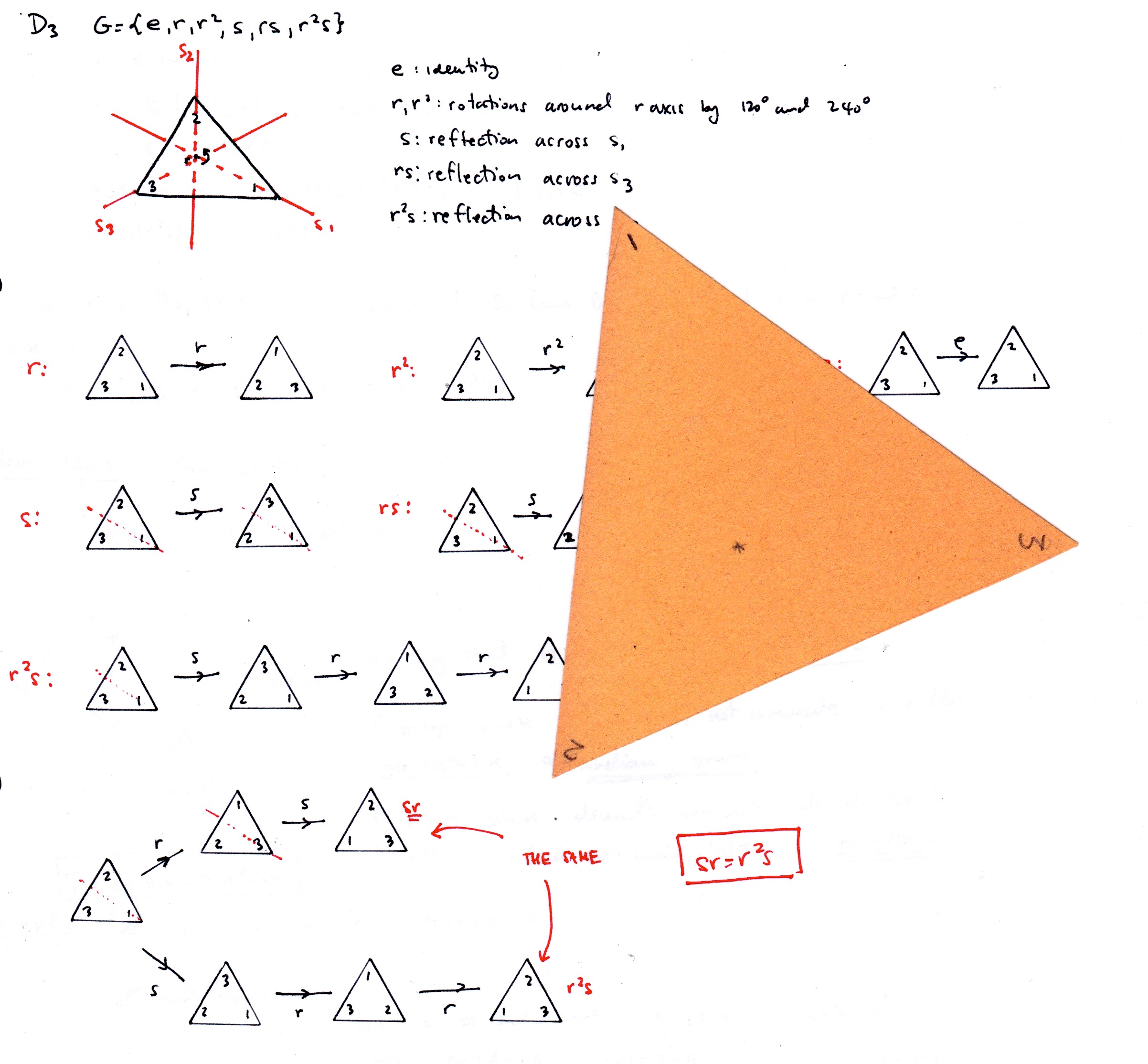
Symmetry transformations of an equilateral triangle.

• We also revisited the already familiar concepts of identity, inverse, generators, group order, and element order, and constructed the Cayley table for D₃.
• We noticed that the order in which rotations and reflections are carried out does matter, which motivated the introduction of the commutativity as a property of two elements. This led us to the definitions of Abelian and a non-Abelian groups.