-
Discovering Group Theory, Day 14
In preparation for the discussion of continuous groups and their applications in physics, we began the class with a short and a bit of a handwaving introduction of the more abstract notion of symmetry, namely that of a symmetry of a physical law. We then moved on to complex numbers, which most students have seen before. After a brief introduction, students practiced working with:
- Complex numbers in the rectangular form
- Operations with complex numbers
- Complex conjugation and modulus
- Complex numbers as points in a complex plane
Finally, students showed that complex numbers form a group under addition, (C,+).
-
Discovering Group Theory, Day 13
On day 13, we reviewed symmetrical groups and introduced Cayley’s theorem. Students then worked out the mapping between the elements of D3 and S3.
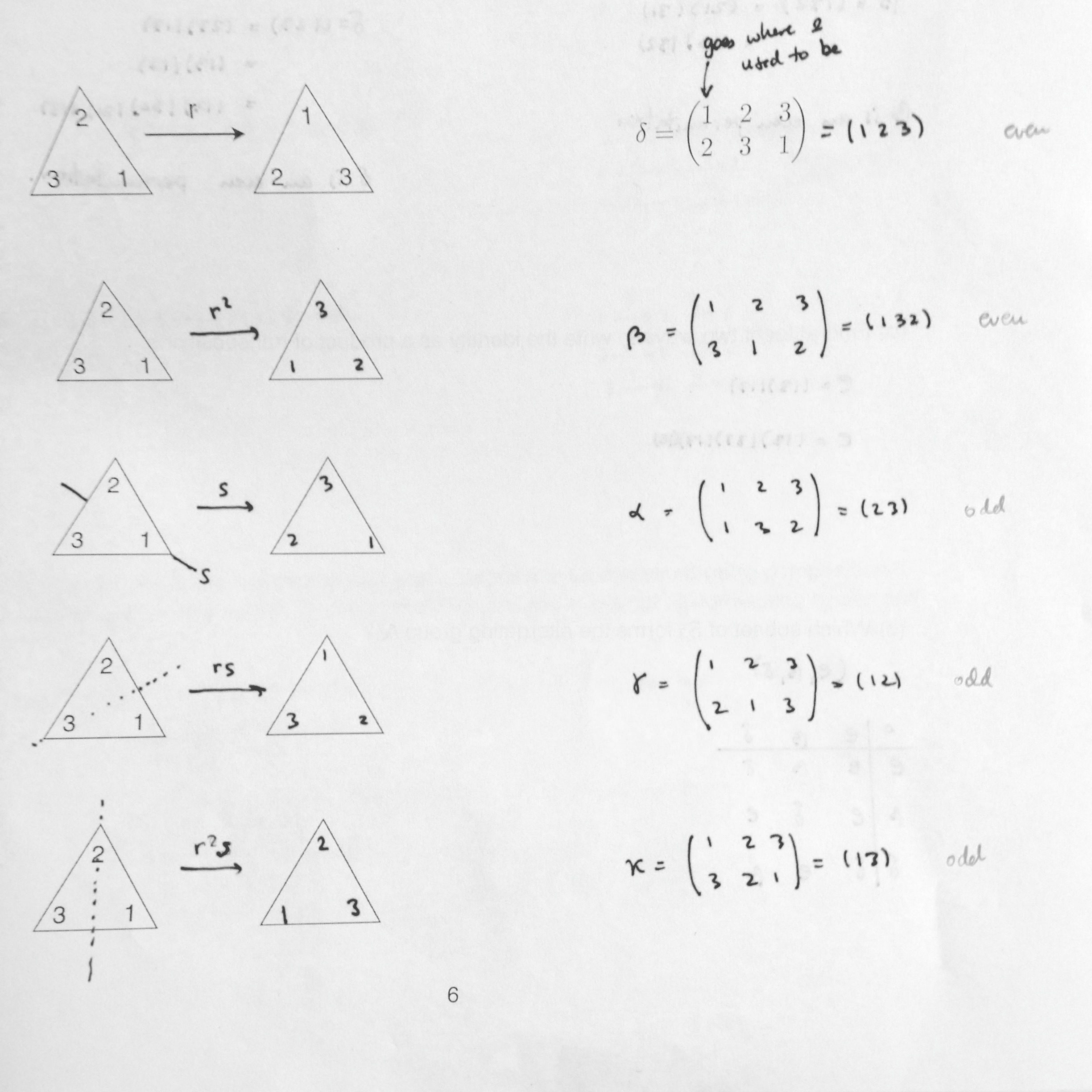
Students explored isomorphism between D3 and S3. -
Color Physics @Hampshire, Day 14
On day 14, in preparation for an activity of making holograms, Prof. Wirth gave a mini lecture on interference of light, its use in early color photography, and its application to holography.
After that, we continued our exploration of subtractive mixing with filters. Students worked through a worksheet in which they had to answer quantitative and qualitative questions. Among other things, students
- Made predictions about the intensity distribution of transmitted light given a wide-spectrum white light incident on ideal filters with a variety of transmittance curves, and compared them with observations.
- Made predictions about the intensity distribution of transmitted light when colored light is incident on ideal filters with a variety of transmittance curves, and compared them with observations.
- Sketched the transmittance curves for a combination filters.
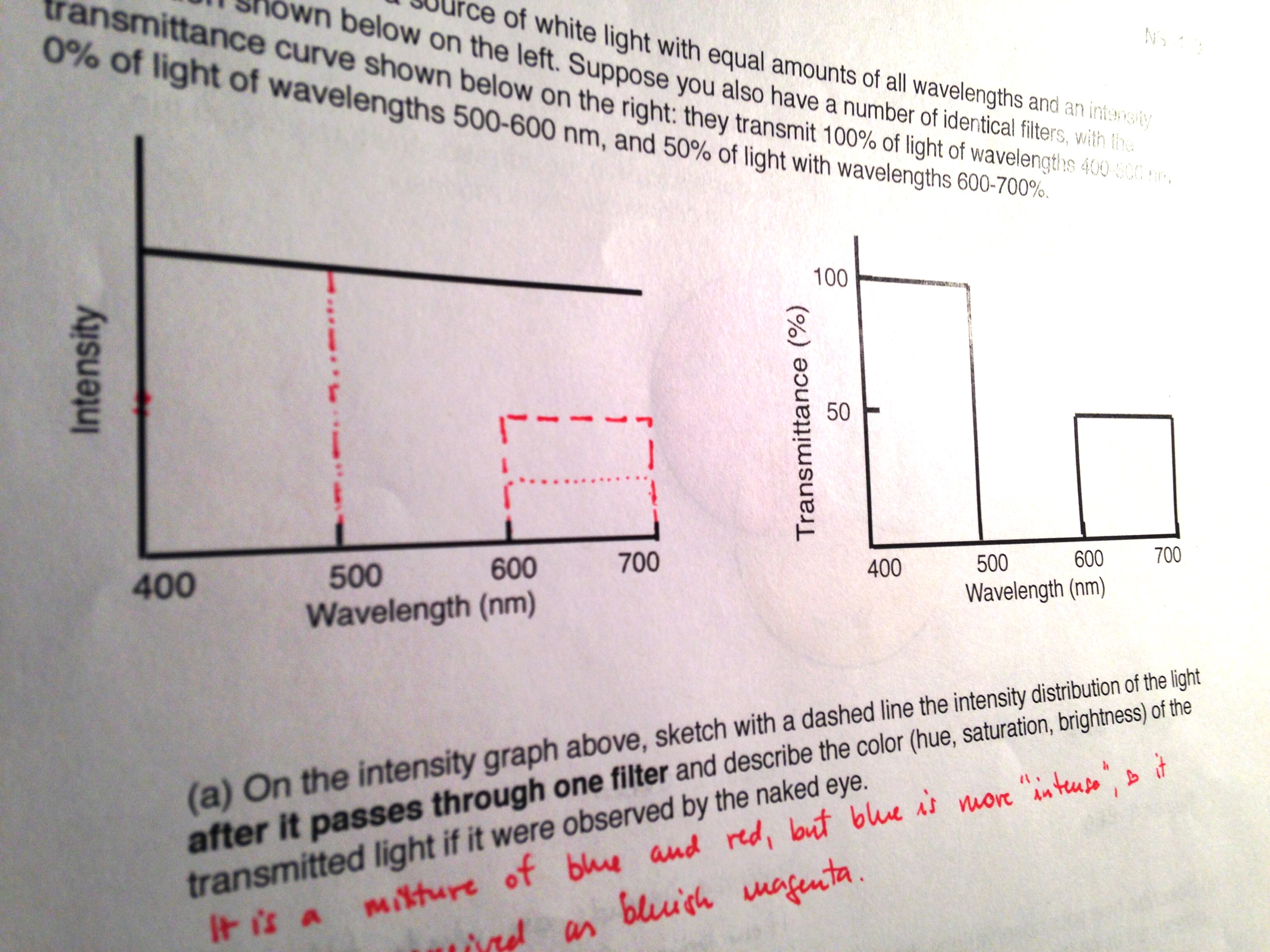
We worked on transmittance curves for multiple filters. - Looked at the real transmittance curves of our filters to understand why not all of the light is blocked by overlapping cyan, magenta, and yellow filters.
At the end, we went over all of the answers as a class.

Subtractive mixing with filters. Materials:
-
Color Physics @Hampshire, Day 12
On day 12 we continued to talk about subtractive mixing with filters. We covered the following topics
- Subtractive mixing with filters
- Transmittance curves
- Ideal vs real fiters
- Cyan, Magenta, and Yellow (CMY) color system
- We also touched on color photography (and in particular the work of the physicist J. C. Maxwell) the early color films, including the two-color system and the three-color Technicolor system. We saw a nice example of early film created using the two-color system, for which the camera had rapidly changing red and green filters.
Other resources:
- An informal on-line resource on the use of subtractive color mixing in early film.
- Malcolm S Longair, “Maxwell and the science of color.” Phil. Trans. R. Soc. A 2008 366 1685-1696; DOI: 10.1098/rsta.2007.2178..Published 28 May 2008.
-
Discovering Group Theory, Day 12
On Day 12, we continued to explore the group of permutations of finite sets using S3 as an example, but we also practiced calculations with elements of symmetric groups of higher orders.

On day 12, more on permutations. In addition to reviewing the concepts of order of an element, inverse, commutativity, we introduced some new concepts:
- Cycles and their properties
- Parity/ Even and odd permutations
Students practiced switching between different notations used to represent permutations, while also checking our answers by physically moving pieces of paper labeled with numbers 1, 2, and 3.

Students carried out permutations of pieces of paper
to either find answers or check them. -
Color Physics @Hampshire, Day 11
Day 11 was more or less a rehash of the second half of Day 10, but in more depth and with more contact points with the visual arts.
The topics covered were:
- Infrared and ultraviolet photography.
- Metamers.
- Missing complementary pairs of spectral colors.
- Three types of additive color mixing and its applications:
- Direct addition of lights (TV monitors and projections on white surfaces in stage design).
- Partitive mixing and pointillism in painting (Seurat, Signac, and van Gogh).
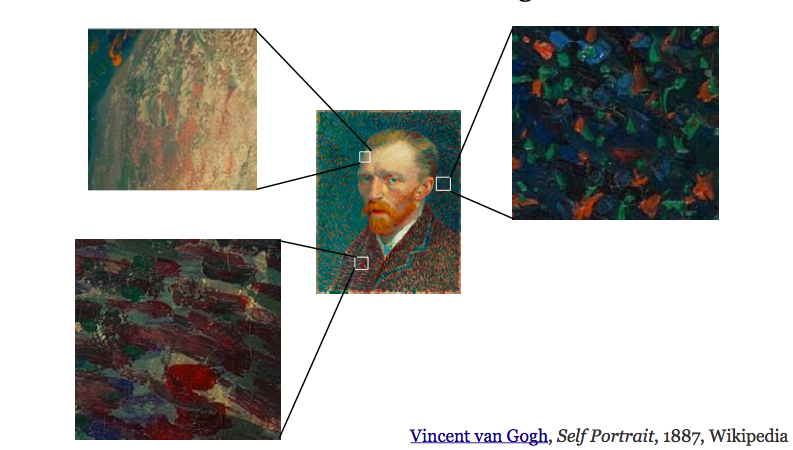
A self portrait of van Gogh’s as an example of the pointillist painting technique which relies on the principle of additive color mixing. - Mixing light “in time” with Newton’s disks, which students made for a homework assignment a few weeks ago.
Finally, we formally introduced subtractive mixing with filters and the transmittance graphs. Students then used diffraction gratings to break up the light from a tungsten lamp, observed which colors were absorbed by various filters, and sketched the transmittance graphs for those filters.

A student observing the color spectrum generated by a diffraction grating after the light has passed through a filter. Other interesting resources:
- Andrew Davidhazy, Overview of Infrared and Ultraviolet Photography, School of Photographic Arts and Sciences Rochester Institute of Technology. Accessed March 11, 2017.
- J. Kirby, K. Stonor, A. Roy, A. Burnstock, R. Grout and R. White. “Seurat’s Painting Practice: Theory, Development and Technology.” National Gallery Technical Bulletin, vol. 24, 2003.
- More pointillist painters on artsy.net
-
Color Physics @Hampshire, Day 10
After reviewing the concepts of wavelength, amplitude, and frequency in general, we discussed the special case of electromagnetic waves and the electromagnetic spectrum. The key ideas covered were the
- Inverse relation between the wavelength and frequency of an electromagnetic wave.
- Intensity of a wave and its relation to the amplitude of the wave.
In a lecture-heavy portion of the class, we went over how different properties of an EM wave in the visible spectrum correlate with the hue, saturation, and brightness of the perceived light. I used very good slides developed by Geoff Boynton at the University of Washington.
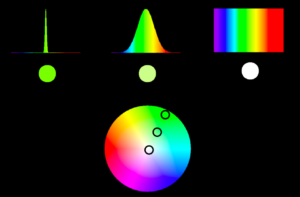
Geoff Boynton’s slide on the relation of the width of the spectral intensity-distribution of light and the perceived saturation. We introduced the spectral intensity-distribution graphs for light sources and the distinction between spectral colors and non-spectral colors, but also reviewed additive color mixing in the context of the intensity-distribution graphs. We touched on the relevance of additive mixing to the pointillist painting movement developed by Seurat.
Students then used hand-held spectrometers to determine the intensity-distribution for the red, green, and blue LED’s we used in additive mixing exercises earlier in the semester. We also tried to determine the spectrum of the mixture various pairs of lights. This was tricky because of the light pollution in the room and the fact that the light from two LED’s was coming from different angles and scattering within the spectrometer.
 Students measured and recorded the intensity distributions of light from LEDs.
Students measured and recorded the intensity distributions of light from LEDs.
Some of the students got to start playing with color filters and explore their absorption spectrum, but more formal introduction was left for the following class.
-
Color Physics @Hampshire, Day 9
Day 9 was a continuation of our exploration of waves. The class time was divided into two main activities:
- Working in groups on conceptual exercises related to waves (drawing waves, ranking them based on various properties, etc.), led by me.
- Carrying out a more systematic study of waves on a slinky, which included quantitative measurements of the speed of a wave pulse, led by my colleague Fred Wirth who has offered to lend a helping hand this semester and who designed and coordinated this portion of the class.
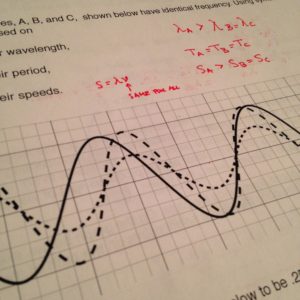
Conceptual Exercises on Waves At the end, we discussed as a class the solutions to some of the conceptual exercises.
-
Color Physics @Hampshire, Day 8
On Day 8, we investigated transverse waves.
- Students did some prep work at home by playing with a PhET interactive simulation exploring qualitatively various properties of a wave on a string, and recording their observations.
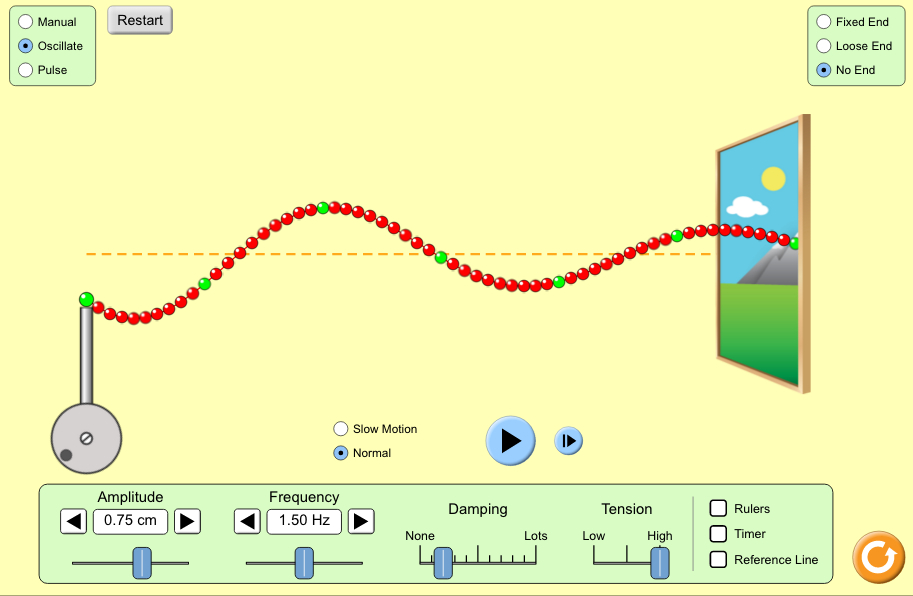
PhET Interactive Simulation on Waves - In class, we introduced a transverse sine wave and students worked in groups to come up with a comprehensive list of properties of a sine wave needed to describe it to grandma. This led us to the concepts of amplitude, wavelength, period, frequency, and speed, as well as their respective appropriate units. We subsequently practiced finding the values of these properties for three animated waves I made using Grapher.
- At the end of the class, as a taste of things to come, students briefly made waves with slinkies.

Making waves with slinkies. - Students did some prep work at home by playing with a PhET interactive simulation exploring qualitatively various properties of a wave on a string, and recording their observations.
-
Discovering Group Theory, Day 9
On Day 9, we learned about subgroups. We motivated the concept of a subgroup using rotational symmetries of regular polygons.
Students began the exploration by finding the symmetry group of a cutout of a dodecagon:- identifying the elements of the group,
- finding the order of the group and the order of each element, and
- writing the Cayley table for the group.
Finding the order of each element proved to be a bit involved because one of the elements is of order 12 (and we have been working with low orders, up to 6 at most). So students explained to each other their methods for quickly finding the order of an element by looking at multiples of 12 and using the fact that an element and its inverse have the same order.
Once we figured out that we are dealing with the cyclic group C12, students were asked to overlay cutouts of various other regular polygons on top of the dodecagon and identify which elements of C12 leave the overlaid polygon also unchanged.

Exploring which rotations of a dodecagon leave an overlaid square unchanged. We organized our observations and discovered that those include C3, C4, and C6, which led to a formal definition of a subgroup and the distinction between a proper subgroup and the trivial group, consisting of the identity element. We also found the generators of these cyclic subgroups and noted that the element of order n generates the subgroup Cn.

Summarizing the findings from the exploration. The observation of the orders of the subgroups led us to the Lagrange’s theorem for finite groups (though we still haven’t formally introduced the distinction between finite and infinite groups).
On Day 10, we will dig deeper into subgroups and clean up our notation.