-
Discovering Group Theory, Day 7
On Day 7, we moved on to the symmetries of a square. Using the same methods as for the equilateral triangle, we got the structure of D4, though we left some derivations for a homework assignment. We then made an educated guess about the structure of the symmetry group of a pentagon, D5, and then generalized to the symmetries of an n-dimensional regular polygon, which led us to the formal definition of Dn.
-
Discovering Group Theory, Day 6
On Day 6, we dove into dihedral group D3, which students have been exploring independently as part of their homework and in the context of the symmetries of an equilateral triangle.
- We tackled the group using three methods:
- physically manipulating a cutout of an equilateral triangle,
- drawing diagrams of various operations, simple (single rotation or reflection) and combined (rotation followed by a reflection, etc.), and
- working out the results of combined operations algebraically.This was an attempt to unify the physical experience of manipulating an object, visually representing the effects of those manipulations on the object, and expressing such operations symbolically.
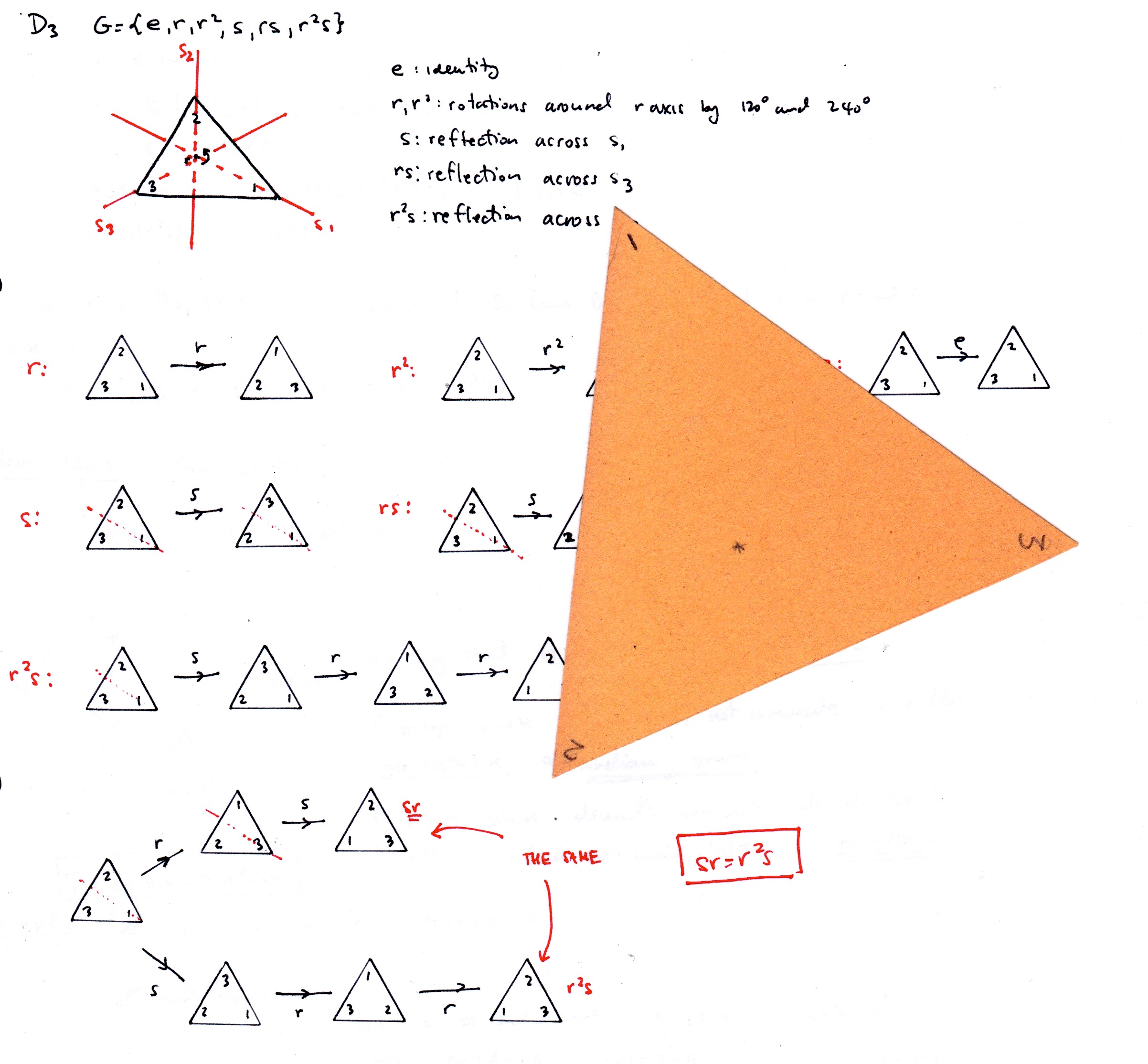
Symmetry transformations of an equilateral triangle.
- We also revisited the already familiar concepts of identity, inverse, generators, group order, and element order, and constructed the Cayley table for D3.
- We noticed that the order in which rotations and reflections are carried out does matter, which motivated the introduction of the commutativity as a property of two elements. This led us to the definitions of Abelian and a non-Abelian groups.
- We tackled the group using three methods:
-
Discovering Group Theory, Day 5
On Day 5, was devoted to the discussion of “Mathematics for Human Flourishing,” a farewell address given by Francis Su at at the end of his term as the President of the Mathematical Association of America.
Students shared their own experiences with mathematics and how those experiences shaped their attitude toward the subject.

An excerpt from Francis Su’s speech. -
Color Physics @Hampshire, Day 4
Day 4 consisted of wrapping up the discussion of HSB, introducing the RGB model, and setting the groundwork for the upcoming discussion of physics.
- Review of of Hue, Saturation, and Brightness
- HSB cylinder
- Additive color mixing: We organized our observations of mixing of colored light from the previous class and introduced the RGB color model.
- Primary colors in additive mixing
- Complementary colors in the additive system
- RBG color model
- RGB cube
- Measurement in physics:
- What is science?
- Units and unit conversions
- Scientific notation and orders of magnitude. We watched Powers of Ten to get a better sense of what different powers of ten mean in terms of different scales, so that we can assess our answers to numerical problems.

Powers of Ten™ Other Resources: A student pointed me to an interactive catalog of objects of a vast range of sizes, organized by powers of ten http://htwins.net/scale2/
- Review of of Hue, Saturation, and Brightness
-
Discovering Group Theory, Day 4
Realizing that there was much to be gained from using now familiar cyclic groups to introduce more group properties, we postponed the exploration of dihedral groups and instead spent the day exploring.
- The review of group properties
- After reviewing the structure of particular examples of cyclic groups (C1, C2, C3, C4, and C6) students extrapolated the properties of Cn, which led us to the formal definition of cyclic groups.
- Modular Addition and (Zn,+n)
- We built on our existing knowledge of how to calculate the hour reading of a clock to motivate modular addition. Students were asked to come up with a general mathematical formula which can be used to do the calculations.
- Working in pairs, students then investigated whether (Z4,+4) forms a group by checking if it satisfies the four axioms and constructing the Cayley table. This exercises broadened our understanding of group “multiplication,” which was originally introduced as a way to represent compounding effects of subsequent rotations of a polygon. It became clear why we have been using quotes around the word multiplication.
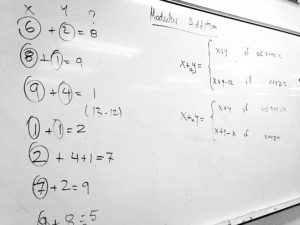
Realizing we already know how to do modular addition. -
- After comparing C4 and Z4, we introduced the idea of a group isomorphism.
- What is Not a Group? Students wanted to know more about when a set and a “product” do not form a group, so we went through a couple of examples in which one or more of the four axioms is violated (especially associativity).
- The review of group properties